Jaap's Puzzle Page

Polydron Enigma

Polydron Enigma

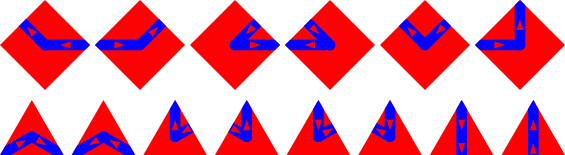
The Polydron Enigma consists of a 6 square and 8 triangular Polydron tiles. Polydron is a brand of construction tiles that can connect along their edges to form a hinge. The 14 tiles of the Enigma join together to form a cuboctahedron. Every tile has a path drawn on one side that goes from one edge or corner to another. The aim of the puzzle is to build the cuboctahedron such that there is one continuous path that visits all its faces and which does not visit any corner more than once.

The tiles are shown below.

Polydron Enigma Tiles

David Singmaster wrote about tile construction sets in Cubic Circular #7/8 (1985), p20-23, and noted that the Polydron tiles were "definitely the nicest that I have seen." The Polydron Enigma was released a few years later. The tiles were invented by Edward Harvey, and patented as GB 1,378,942, published on 2 January 1975. He also designed the Polydron Enigma.

The number of positions:

The puzzle's 6 squares can be ordered in 6! ways, and rotated in 46 ways. Similarly, the triangles have 8! orderings and 38 orientations. The tiles are all distinct and have not rotational symmetry. Therefore they can be put together in 6!·8!·46·38 ways. The cuboctahedron has 24 orientations, so number of distinct completed cuboctahedra that can be made is 6!·8!·46·38 / 24 = 32,506,616,217,600.
This is of course not a realistic measure of difficulty, since you would not continue placing tiles to the end after one tile is mismatched.

Links to other useful pages:

Polydron homepage. Although the Polydron Enigma is no longer made, construction sets of tiles in various colours are available.

Solution:

I have run a computer search to find all solutions. It found 218 where all the edges matched, and no corner is visited more than once. Many of these however consist of two or more loops rather than a single long loop. After weeding those out, only 81 single loop solutions remain.

Polydron Enigma Solution 01 Polydron Enigma Solution 02 Polydron Enigma Solution 03 Polydron Enigma Solution 04 Polydron Enigma Solution 05 Polydron Enigma Solution 06 Polydron Enigma Solution 07 Polydron Enigma Solution 08 Polydron Enigma Solution 09 Polydron Enigma Solution 10 Polydron Enigma Solution 11 Polydron Enigma Solution 12 Polydron Enigma Solution 13 Polydron Enigma Solution 14 Polydron Enigma Solution 15 Polydron Enigma Solution 16 Polydron Enigma Solution 17 Polydron Enigma Solution 18 Polydron Enigma Solution 19 Polydron Enigma Solution 20 Polydron Enigma Solution 21 Polydron Enigma Solution 22 Polydron Enigma Solution 23 Polydron Enigma Solution 24 Polydron Enigma Solution 25 Polydron Enigma Solution 26 Polydron Enigma Solution 27 Polydron Enigma Solution 28 Polydron Enigma Solution 29 Polydron Enigma Solution 30 Polydron Enigma Solution 31 Polydron Enigma Solution 32 Polydron Enigma Solution 33 Polydron Enigma Solution 34 Polydron Enigma Solution 35 Polydron Enigma Solution 36 Polydron Enigma Solution 37 Polydron Enigma Solution 38 Polydron Enigma Solution 39 Polydron Enigma Solution 40 Polydron Enigma Solution 41 Polydron Enigma Solution 42 Polydron Enigma Solution 43 Polydron Enigma Solution 44 Polydron Enigma Solution 45 Polydron Enigma Solution 46 Polydron Enigma Solution 47 Polydron Enigma Solution 48 Polydron Enigma Solution 49 Polydron Enigma Solution 50 Polydron Enigma Solution 51 Polydron Enigma Solution 52 Polydron Enigma Solution 53 Polydron Enigma Solution 54 Polydron Enigma Solution 55 Polydron Enigma Solution 56 Polydron Enigma Solution 57 Polydron Enigma Solution 58 Polydron Enigma Solution 59 Polydron Enigma Solution 60 Polydron Enigma Solution 61 Polydron Enigma Solution 62 Polydron Enigma Solution 63 Polydron Enigma Solution 64 Polydron Enigma Solution 65 Polydron Enigma Solution 66 Polydron Enigma Solution 67 Polydron Enigma Solution 68 Polydron Enigma Solution 69 Polydron Enigma Solution 70 Polydron Enigma Solution 71 Polydron Enigma Solution 72 Polydron Enigma Solution 73 Polydron Enigma Solution 74 Polydron Enigma Solution 75 Polydron Enigma Solution 76 Polydron Enigma Solution 77 Polydron Enigma Solution 78 Polydron Enigma Solution 79 Polydron Enigma Solution 80 Polydron Enigma Solution 81