Tantrix

Introduction

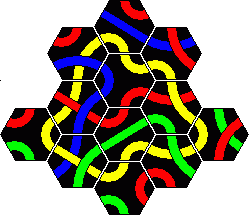
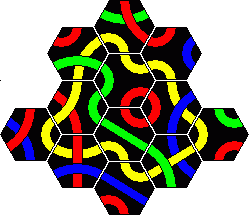
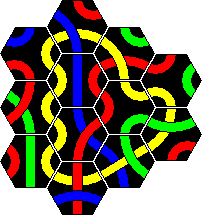
Tantrix is the brand name for a set of hexagonal Bakelite tiles. Each tile has three lines, of different colours, which go from one side to another. Some sets of tiles can be use as puzzles, in which you have to arrange the tiles so that the edges match in colour, thus forming coloured lines or even loops. The tiles can also be used for playing a game with two or more people. On this page however, I will only discuss the puzzles.

There are three kinds of line segments - straights, bends, and corners - and these lead to four different types of Tantrix tile:

Three corners

A straight and
two corners

A straight and
two (shallow) bends

A corner and
two bends

The fifth possible tile shape, a tile with three straights, is not used in Tantrix.

The Tantrix game and puzzles were invented by Mike McManaway. The Tantrix tiles seem to be based on an older tile set, created some 40 years earlier by Charles Titus and Craige Schenstedt. It was marketed then as Psychepaths, but is now made and sold by Kadon Enterprises, Inc. with the name Kaliko. The Kaliko tiles form a complete set of all possible path patterns with 3 or fewer colours. Their patterns differ from Tantrix only in that a colour may be repeated on a tile, and the tile type with 3 straights is used as well.

There are and have been several different Tantrix tile sets available. There are various small sets, each containing about 10 distinct tiles, with which there are one or more puzzles to solve. There is however also a complete set of 56 tiles, and from that any of the small sets can be selected.

Each tile uses exactly 3 colours. There are 14 possible tiles with three colours: 2 with three corners, 3 with a straight and two corners, 3 with a straight and two bends, and 6 with a corner and two bends. The full set of tiles uses four colours (red, blue, green , yellow). Each tile is therefore missing exactly one of the four colours. This means that there are 4 sets of 14 tiles, for a total of 56 tiles.

I acquired the full set of 56 tiles quite a few years ago. At that time the numbering of the tiles was given in the accompanying booklet. Nowadays a different numbering scheme is used, and the numbers are engraved on the backs of the tiles and those numbers are painted in 5 different colours.

If we use the letters R, B, G, and Y for the path colours (red/blue/green/yellow), then the pattern on a tile can be described by a list of six such letters which denote the colour at each of the edges, going clockwise around the tile. Below are conversion tables for the two tile numberings, also listing all the tile patterns, and the colour of the number in the new numbering.

Old	New	Colour
37	1y	YYBRBR
33	2y	RRBYYB
29	3y	BBYYRR
36	4r	BRYBYR
32	5r	BBRYYR
34	6b	YBRYRB
42	7b	BBYRYR
41	8b	BBRYRY
35	9y	RBYRYB
38	10r	YYRBRB
39	11r	RRBYBY
40	12y	RRYBYB
31	13b	BBYRRY
30	14b	BBRRYY

5	15r	GGRYYR
3	16r	GGYRRY
10	17y	YYGRGR
9	18r	YYRGRG
13	19r	RRGYGY
14	20y	RRYGYG
1	21y	GGRRYY
4	22y	RRGYYG
2	23y	GGYYRR

47	24r	GGBRRB
43	25r	BBGGRR
45	26r	BBGRRG
53	27r	RRBGBG
44	28r	BBRRGG
54	29r	RRGBGB
46	30r	BBRGGR

11	31y	GGRYRY
7	32g	GRYGYR
12	33g	GGYRYR
8	34g	RGYRYG
6	35g	YGRYRG

52	36g	GGRBRB
56	37g	BBGRGR
55	38g	BBRGRG
48	39b	GBRGRB
50	40b	BGRBRG
51	41b	GGBRBR
49	42b	RBGRGB

15	43b	BBYYGG
20	44y	YBGYGB
16	45g	BBGGYY
25	46g	GGBYBY
17	47g	BBYGGY
19	48w	GGBYYB
18	49w	BBGYYG
22	50w	BGYBYG
21	51w	GBYGYB
26	52w	GGYBYB
24	53w	YYGBGB
23	54w	YYBGBG
27	55w	BBGYGY
28	56w	BBYGYG

Old	New	Colour
1	21y	GGRRYY
2	23y	GGYYRR
3	16r	GGYRRY
4	22y	RRGYYG
5	15r	GGRYYR
6	35g	YGRYRG
7	32g	GRYGYR
8	34g	RGYRYG
9	18r	YYRGRG
10	17y	YYGRGR
11	31y	GGRYRY
12	33g	GGYRYR
13	19r	RRGYGY
14	20y	RRYGYG

15	43b	BBYYGG
16	45g	BBGGYY
17	47g	BBYGGY
18	49w	BBGYYG
19	48w	GGBYYB
20	44y	YBGYGB
21	51w	GBYGYB
22	50w	BGYBYG
23	54w	YYBGBG
24	53w	YYGBGB
25	46g	GGBYBY
26	52w	GGYBYB
27	55w	BBGYGY
28	56w	BBYGYG

29	3y	BBYYRR
30	14b	BBRRYY
31	13b	BBYRRY
32	5r	BBRYYR
33	2y	RRBYYB
34	6b	YBRYRB
35	9y	RBYRYB
36	4r	BRYBYR
37	1y	YYBRBR
38	10r	YYRBRB
39	11r	RRBYBY
40	12y	RRYBYB
41	8b	BBRYRY
42	7b	BBYRYR

43	25r	BBGGRR
44	28r	BBRRGG
45	26r	BBGRRG
46	30r	BBRGGR
47	24r	GGBRRB
48	39b	GBRGRB
49	42b	RBGRGB
50	40b	BGRBRG
51	41b	GGBRBR
52	36g	GGRBRB
53	27r	RRBGBG
54	29r	RRGBGB
55	38g	BBRGRG
56	37g	BBGRGR

Click image to see larger version

Original Tantrix Puzzle (Crazy Tantrix)

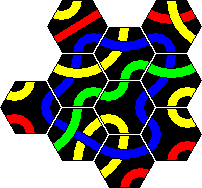
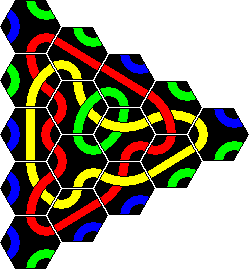
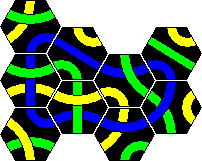
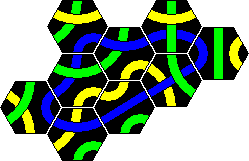
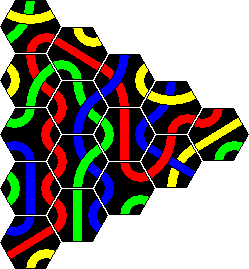
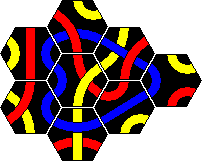
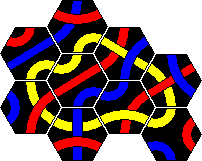
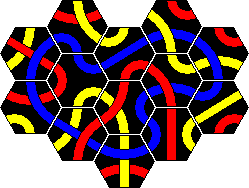
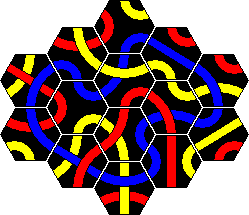
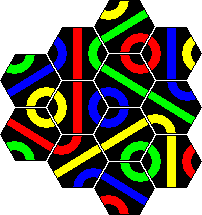
The first version of Tantrix was a puzzle consisting of 10 hexagonal tiles. The aim is to tile the pieces (without leaving holes) to make a single loop using all the parts of one colour and such that the edges of all adjacent tiles match colours. Any colour loop is possible. It is therefore three puzzles in one, one for each colour.

The tiles are shown below. I have shown them using the colours red, blue, and yellow. The colours of the real sets did vary however. I got one set with the aforementioned colours. For a present I received second set of 10 Tantrix pieces, but these used green instead of yellow. Other than that they are exactly the same.

BBRRYY
(30|14b)

BBRYYR
(32|5r)

BBYRRY
(31|13b)

BRYBYR
(36|4r)

RBYRYB
(35|9y)

YBRYRB
(34|6b)

BBRYRY
(41|8b)

BBYRYR
(42|7b)

YYBRBR
(37|1y)

YYRBRB
(38|10r)

The solutions of the red/blue/yellow loops are shown further down the page. You can click the link below-right to jump there directly.

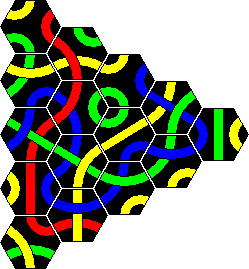
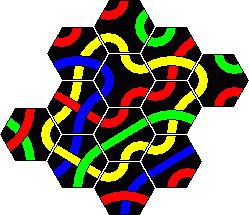
It is possible to combine two differently coloured Crazy Tantrix sets to make a larger puzzle. I have combined my red/blue/yellow and red/blue/green sets to make a large red loop. A blue loop is also possible, though more difficult. A solution to the red loop is given in the solutions section.

Solutions to the original puzzle
Tips on solving loop puzzles

About the background of this page

The background image used on this page consists of a repeated pattern using the ten tiles of the Original Puzzle. It was quite difficult to find a ten-tile shape that can be tiled easily. I do not believe that there is such a shape that tiles the plane with only translations, and that only shapes that need rotations as well are possible. What makes it harder is that the background image must be rectangular. In the end I plumped for the triangle shape shown below that combined with an upside down copy of itself forms a 4 by 5 diamond. By making sure its edges were such that the diamond could be tiled in a regular rectangular manner, the eventual background image would remain fairly small. Click the image to see the rectangular repeated pattern. I found several solutions, but this one is the only one I found that has infinitely long lines of all three colours, though it does have a loop. I did not find any with only loops or only lines that use ten tiles, but have not done an exhaustive search.

I did find a very nice 9-tile shape (3x3 diamond) that tiles the plane (without rotations) and has only loops, and another that has only lines. The trouble with these however is that they do not tile in a rectangular way, so the background image would have to be quite large and contain many copies if this shape (18 in fact), or be skewed in some way. The tiling patterns are shown below. Click on them to see the large rectangular tile that would be needed for a background.

Tantrix Xtreme Puzzle

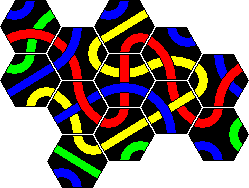
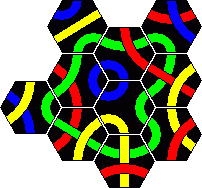
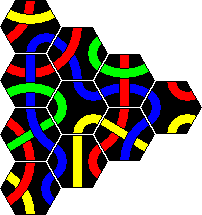
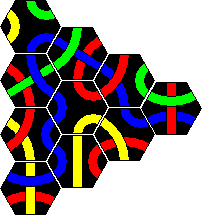
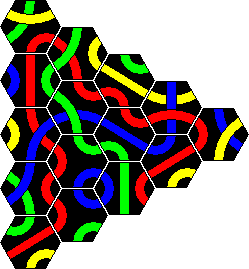
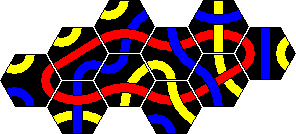
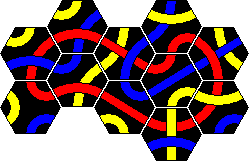
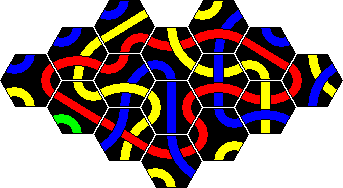
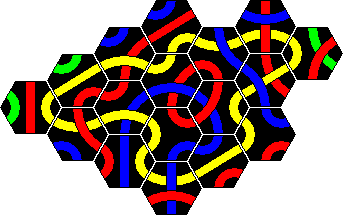
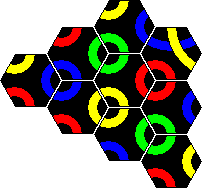
Tantrix Xtreme is a puzzle version of Tantrix similar to the original, but more challenging. It also consists of 10 hexagonal Tantrix tiles, but now four colours are used in the set, and the tiles are numbered on the back. The tiles are shown here:

Tile 1
BBRGRG
(55|38g)

Tile 2
BBRGGR
(46|30r)

Tile 3
BBGGRR
(43|25r)

Tile 4
BGRGBR
(49|42b)

Tile 5
BBGRRG
(45|26r)

Tile 6
RGBGRB
(50|40b)

Tile 7
RRBGBG
(53|27r)

Tile 8
RRYBYB
(40|12y)

Tile 9
BRYRBY
(34|6b)

Tile 10
BBYRYR
(42|7b)

Note that the exact colours may vary. In my set it is more purple than red, and uses white instead of yellow. For consistency I have used the four standard colours on this page however.

There are all together 10 challenges, from easy to rather hard. Start with the tiles numbered 1-3, and make a single loop of one colour. The same rules apply as with almost all other Tantrix puzzles - all adjacent tile edges should match colour, and there should not be holes in the tile arrangement. Once you have done a loop with tiles 1-3, then try to make a loop with 1-4, and then 1-5 and so on until you eventually use all ten tiles to build a loop.
In most cases only one of the colours can be used for making the loop, the others being impossible. In some cases, in particular the 10 tile loop, more than one colour loop is possible.

After those eight loop challenges, there are two difficult line puzzles. The aim is to put the ten tiles in a triangle shape, but with the red or the blue colour forming one long line through all the tiles. Both red and blue have solutions.

Solutions to the Xtreme Tantrix
Tips on solving loop puzzles

The Super 5 Puzzles

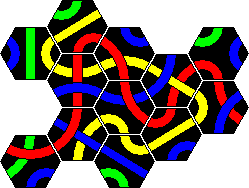
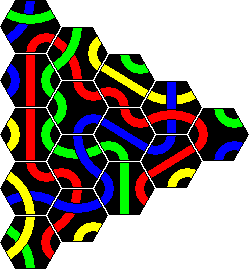
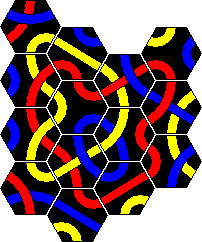
In the booklet that comes with the 56 tile set several puzzles are given. The tiles can be separated into 5 separate sets, which are called the 'Super 5' puzzles. They are called Junior, Student, Professor, Master, and Genius. Apparently these were sold separately as well.

In the Junior, Student and Master puzzles the aim is to make a loop of one colour, just like the Original puzzle. Of course, the loop must include all the tiles with that colour, and the tiles may not enclose a hole. In the Professor puzzle the aim is to make two simultaneous loops. Again each loop must use all the tiles of that colour. Tiles which have both chosen colours must therefore be incorporated in both loops. The Genius puzzle is extremely difficult, as now you must make two lines instead of loops. The restrictions that loops impose do not apply to this puzzle. It has two types of solutions (red/blue, red/yellow), and it took me about a week to find a solution for each. I thought there was essentially only one solution of each type, but Alexander Fronk sent me a second solution for the red/yellow lines.

Solutions to Super 5 puzzles

Three Pyramid Puzzles

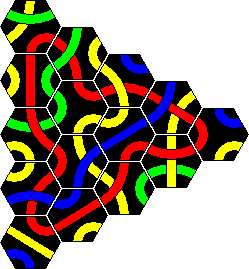
The booklet of the Tantrix game used to contain three pyramid puzzles. With these you have to use 15 tiles and they all have solutions in a pyramid shape, i.e. a size 5 triangle. In puzzle 1 you have to make a red loop, in puzzle 2 two loops using any two colours (like the Super 5 Professor puzzle), and in puzzle 3 two lines of any two colours (like the Super 5 Genius puzzle). Puzzle 3 is extremely hard to solve. The current booklet no longer contains these puzzles. The tiles to use are listed below:

Solutions to the Pyramid Puzzles

The Rainbow Puzzles

Nowadays another set of puzzles is given. Like the Super 5 puzzles there are five puzzles that together make the full set of 56 tiles. The numbers engraved on the back of the tiles are painted in 5 colours, so that they can easily be separated into the 5 sets of tiles used. The puzzles are as follows:

Solutions to the Rainbow Puzzles

The Tantrix Discovery Puzzles

The Tantrix Discovery is very much like Tantrix Xtreme. It is a set of 10 tiles with which you can do several loop puzzles. They are exactly the tiles numbered 1 to 10 in the complete set (as it is sold now, i.e. the new numbering scheme). Start with the tiles numbered 1-3, which are yellow numbers, and make a single yellow loop. Then add tile number 4, which is a red number, and try to make a red loop. Continue on in the same way, each time adding the next tile and trying to make a loop of the colour which that number has.

If you have more than one Discovery set then you can combine them to make larger loops, again adding individual tiles in numerical order and making a loop of the colour given by colour of the tile number just added. This can continue to indefinitely large loops, but I will not give solutions to that.

The current booklet with the full set also says that you can continue by adding tiles from 11 onwards, all the way up to 30. In the solutions section you can find a solution for each of these loops. For completeness, here are the tiles used in the Discovery puzzles from the full set:

Solutions to the Discovery Puzzles

The "Unsolved" Puzzles:

There are two so-called "unsolved" puzzles, using all 56 tiles.

1. The first is to find an arrangement with the four longest lines, one of each colour. Only the longest line of each colour counts, and the best is the one with the longest total length. The current record according to the Tantrix homepage is 146=40+37+35+34. Note that since all possible tiles are in the set, any solution can be rearranged such that the colours are swapped around.

It can be proved that 146 is the maximum attainable, so this puzzle has actually been solved. Nobody has ever done so by hand without computer assistance.
Sketch of proof: Consider an arrangement with 4 lines of total length 146. A line of length n uses n tiles, and therefore involves 2n tile sides. Of these, 2n-2 sides are internal, and 2 sides are the endpoints. The 4 lines will therefore use 2·146-8=284 internal sides of tiles. The 56 tiles have 6·56=336 sides all together, leaving 336-284=52 sides along the outside of the arrangement. Longer lines would leave fewer external sides. The size 5 regular hexagon with one edge shaved (i.e. a hexagon with sides 5, 5, 5, 4, 6 and 4) is the shape with the smallest possible perimeter, namely 52. Therefore the lines cannot be longer than 146.
The difficult step is proving that the hexagon shape has the smallest perimeter. I have proved this, in a long and tedious manner as follows: first show that the best shape is nearly convex, i.e. has at most one tile with one external edge. Then write the number of tiles in each row in a list, and show that with the best shape the list will look something like this: 7, 8, 9, 10, 9, 8, 5, i.e. first strictly increasing then decreasing and with successive differences equal to 1 except possibly for the last one. This leaves only a relatively small number of possibilities, and of these the sequence 6, 7, 8, 9, 10, 9, 8, 7, 6, 5 is the best.

2. The second unsolved puzzle is to find the arrangement with the four longest loops. The current record is 136=38+35+33+30. In 2003 Jack Kuipers became the first person to solve this puzzle by hand without using a computer.

It can probably be proved that 136 is the maximum attainable.
Sketch of proof:Any shape with 56 tiles must have some tiles with an odd number of external sides, because they cannot be arranged in a triangle (tiles with 4 external edges are at a 60 degree corner, with 2 external edges along a straight side of the arrangement). Each tile with an odd number of external sides must have at least one line connecting to an internal with an external side. Thus any such odd corner will be the start of a line leading into the arrangement, wasting internal sides which are better used for forming loops. The best shape is therefore one with a small perimeter, but which has few odd corners and has its odd corners close together.
Consider a size 10 triangle with one extra tile added anywhere on its side. This has perimeter 62, and its two odd corners are adjacent so these waste only 2 internal sides. This leaves 336-64=272 internal sides for a total loop length of 272/2=136. Any other shape with more odd corners that is more convex will have a smaller perimeter but all internal sides gained are probably all wasted on the lines between the odd corners. It is likely that no other shapes have more available internal sides.
Again the difficulty is proving that this shape is the best. I have not properly proved this.

Solutions to the "Unsolved" Puzzles

My own puzzles

The Master puzzle above (see the Super 5 Puzzles) has many solutions. I found about 30 solutions in one evening. The loop in a solution has both convex and concave parts. Usually three tiles are involved in the concave parts (the 'dents' if you like). As far as I know there is only one solution in which only two tiles are part of the concave sections of the loop. Find it.
From the full set, take the 12 pieces which have a straight line and two bends. Try to make the shape shown on the right - a triangle with sides of length 5 but with its tips missing. In other words, the shape with rows of lengths 2,3,4,3.
Do the same as the previous puzzle, but with the 12 pieces which have a straight line and two corners.
Take the 8 pieces which have 3 corners, and any single piece with a corner and two bends (e.g. RRBYBY). Try to make any shape containing 5 circles.
The unsolved line problem can also be done using only three colours. In other words, using the 14 tiles with only three of the colours make a shape with the longest three lines. Only the longest line of each colour counts, and the aim is to make the sum of the three lengths as large as possible. The highest possible total length is 32, and I have two solutions to it.
The unsolved loop problem can also be done using only three colours. In other words, using the 14 tiles with only three of the colours make a shape with the longest three loops. Only the longest loop of each colour counts, and the aim is to make the sum of the three lengths as large as possible. My best solution had total length 8+8+7=23, but I received a solution by Neil O'Rourke with length 12+9+4=25. I don't know what the highest possible total is.

Solutions to my own puzzles

Tips on finding a solution to any loop puzzle:

Before you read on, please be aware that these tips will make some of the puzzles quite easy and may spoil your enjoyment of the puzzle.

First decide which colour you are going to make the loop from. If you are doing the original puzzle, any colour can be solved. The Super 5 puzzles Junior/Student/Master (see below) only allow one colour to be looped. It is usually not hard to decide which colour to use by eliminating those that are impossible.
1. If two colours cross an odd number of times, neither colour can be looped. This is because a colour that crosses into a loop must exit it somewhere
2. If a colour has an odd number of shallow bends it cannot be looped. If you imagine travelling around a loop, your heading will change through 360 degrees. A corner will change your heading by 120 degrees. A shallow bend however changes your heading by 60 degrees so you must pass an even number of them to get your heading to be a multiple of 120, such as the 360 needed for a full circle.
A good strategy is to make a loop of your chosen colour, completely ignoring the other colours. Then swap pairs of pieces that leave the loop intact but fix the other lines so that the colours match up. The following tips delve deeper into this strategy.
Try to fix the lines inside the loop first, and only then the parts on the outside.
If you are doing a puzzle that uses only three colours, remember that every tile uses every colour. In the original Crazy Tantrix for example yellow crosses with blue on only two tiles. Therefore when making a blue loop, a yellow line must enter and exit the loop using the two crossing tiles available and go through every tile that lies mostly inside the loop. Try to arrange the entrance and exit tiles of the yellow line to make it as easy as possible to achieve this.
Once you have a rough idea of where the entrance/exit points of the line should be, try to swap other tiles of the loop to build the line between the two points. Focus only on keeping the loop in the same shape, and building this line across it. If it fails, you may have to move your entrance/exit points and try again. When the loop and the line across it are done, the third and final colour must automatically also be correct inside the loop.
Once the loop and its insides are correct, make swaps of tiles which lie mostly outside the loop to try to correct any faults until all tiles match up.
If you make a red loop with the Crazy Tantrix puzzle, there are four yellow and four blue crossings. In this case choose one of the two colours, and plot two lines that go into and out of the loop, and that together go through every tile that lies mostly inside the loop. Similarly, if you are doing a loop puzzle with four colours on the tiles, look at each colour inside the loop individually.
Making a loop of one colour is relatively simple if you don't care about matching the other colours. You may however wish to explore the many possible loop shapes systematically.
1. First determine the amount of turn that is available in your chosen colour. The bends contribute 1 to the turn and corners 2. Straights are ignored. For example, in the Crazy Tantrix there are 4 blue bends and 5 blue corners. This gives a turn of 4+2×5 = 14. This number represents the amount your heading changes (in units of 60 degrees) in either direction if you were to travel along a path consisting of all those pieces.
2. Subtract 6 to get the excess amount of turn. If you travel around a loop, the net change in your heading is 360 degrees. This therefore requires you to use up 6 from the amount of turn available.
3. Divide by 2 to get the concavity. The excess amount of turn must cancel itself out, which means that half of it is used to turn in one direction, the other half in the other direction. So half of the excess turn is turning against the direction of the loop, and therefore it is the amount of concavity in the loop. It represents how many dents there are in the loop. For example, the Crazy Tantrix blue loop has a concavity of (14-6)/2 = 4. In tip 1 it was already said that there must be an even number of bends for a loop to be possible, and in that case the concavity will be a whole number.
4. Decide how many bends and corners you will use to make up this concavity, where corners count as 2 and bends as 1. A concavity of 4 can be achieved in three ways: using 4 bends, or 2 bends and a corner, or 2 corners. In the case of the Crazy Tantrix blue loop the first of these is easily found to be impossible, because the 4 concave bend tiles must lie mostly inside the loop and there are not enough other blue loop parts to stretch around them. The other two choices do both lead to solutions.
5. Put the concave tiles together in all possible ways (ignoring the other colours for now as usual), and complete the loop around them with the other tiles. In this way you can find many possible loop shapes fairly easily. Be careful not to overlook some loop shapes where the concave tiles don't all touch each other.

Solutions to Original Puzzle

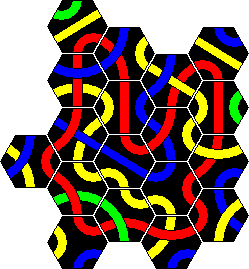
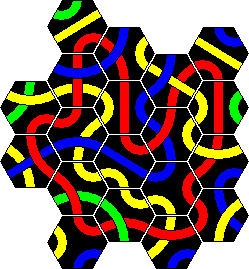
Here are three solutions of the original Tantrix puzzle, one of each colour. There are dozens of possibilities for each, so these are only examples.

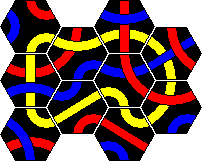
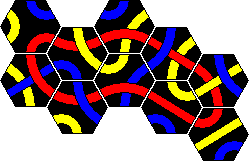
Here is a solution for a large red loop with two sets combined. A blue loop is a little more difficult, and I leave that to you.

Solutions to Tantrix Xtreme

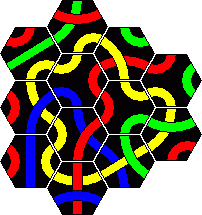
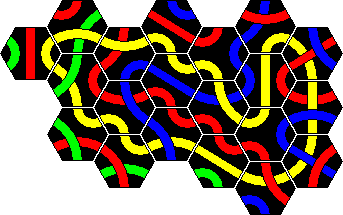
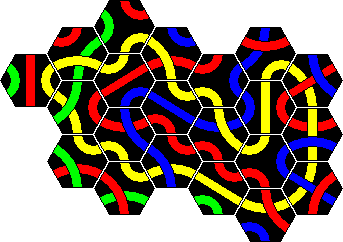
Here are solutions to the 8 loop puzzles. Two solutions are given for the 7 and the 10 tile loop, because two colours are possible. In all the other cases, only one of the colours can be used to make a loop.

1-3, blue
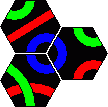
Xtreme 3 tile blue loop

1-4, green
Xtreme 4 tile green loop

1-5, green
Xtreme 5 tile green loop

1-6, red
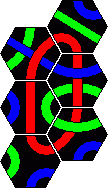
Xtreme 6 tile red loop

1-7, green
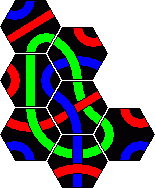
Xtreme 7 tile green loop

1-7, red
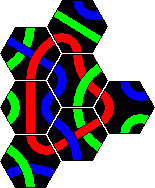
Xtreme 7 tile red loop

1-8, red
Xtreme 8 tile red loop

1-9, blue
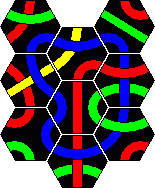
Xtreme 9 tile blue loop

1-10, red
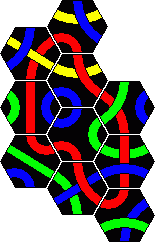
Xtreme 10 tile red loop

1-10, blue
By Michael Gegenwart
Xtreme 10 tile blue loop

Here are the solutions to the 2 pyramid puzzles. There are essentially three solutions for a red line and only one for a blue line. These are shown below.

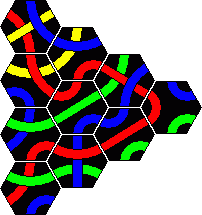
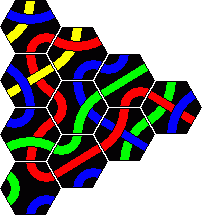
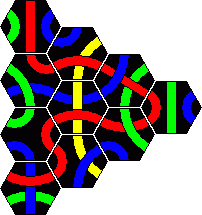
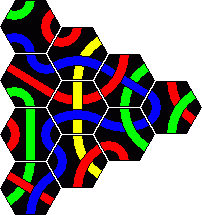
Minor variations are possible by rearranging one or more sets of tiles. For example in the first solution you could swap tiles 2 and 4, or swap tiles 5 and 7, or turn tile 1 around a bit, which leads to 8 variations. In solution 2 only tiles 3 and 8 on the corners can be trivially swapped. In solution 3 we can swap 3 and 5 as well as the trivial corners 2 and 6. There are six variations of the blue solution by rearranging the tiles from left and right columns (tiles 1-5).